How To Find One Side Of A Triangle
Finding a Side in a Right-Angled Triangle
Find a Side when nosotros know some other Side and Angle
We can find an unknown side in a right-angled triangle when we know:
- one length, and
- ane angle (apart from the right angle, that is).
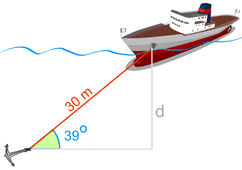
Example: Depth to the Seabed
The send is anchored on the seabed.
We know:
- the cable length (30 grand), and
- the angle the cable makes with the seabed
So we should exist able to find the depth!
Just How?
The reply is to use Sine, Cosine or Tangent!
Only Which I?
Which one of Sine, Cosine or Tangent to use?

To discover out which, first we give names to the sides:
- Adjacent is next (next to) to the bending,
- Opposite is opposite the angle,
- and the longest side is the Hypotenuse.
Now, for the side nosotros already know and the side we are trying to detect, we use the get-go letters of their names and the phrase "SOHCAHTOA" to make up one's mind which part:
| SOH... | Sine: sin(θ) = Opposite / Hypotenuse | |
| ...CAH... | Cosine: cos(θ) = Adjacent / Hypotenuse | |
| ...TOA | Tangent: tan(θ) = Opposite / Adjacent |
Like this:


Instance: Depth to the Seabed (Continued)
Find the names of the two sides we are working on:
- the side nosotros know is the Hypotenuse
- the side we want to notice is Reverse the angle (check for yourself that "d" is opposite the bending 39°)
Now use the first letters of those two sides (Opposite and Hypotenuse) and the phrase "SOHCAHTOA" which gives united states "SOHcahtoa", which tells us we need to use Sine:
Sine: sin(θ) = Opposite / Hypotenuse
Now put in the values we know:
sin(39°) = d / 30
And solve that equation!
Simply how do we summate sin(39°) ... ?
 | Employ your calculator. |
sin(39°) = 0.6293...
And so now we have:
0.6293... = d / 30
Now nosotros rearrange it a niggling bit, and solve:
Showtime with: 0.6293... = d / 30
Bandy sides: d / 30 = 0.6293...
Multiply both sides by xxx: d = 0.6293... x 30
Calculate: d = eighteen.88 to ii decimal places
The depth the ballast band lies below the pigsty is xviii.88 m
Stride Past Pace
These are the four steps to follow:
- Step 1 Find the names of the ii sides we are using, one nosotros are trying to find and one we already know, out of Opposite, Adjacent and Hypotenuse.
- Step two Utilize SOHCAHTOA to determine which one of Sine, Cosine or Tangent to use in this question.
- Footstep iii For Sine write down Opposite/Hypotenuse, for Cosine write down Adjacent/Hypotenuse or for Tangent write down Opposite/Adjacent. Ane of the values is the unknown length.
- Step four Solve using your figurer and your skills with Algebra.
Examples
Let's wait at a few more examples:
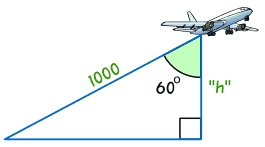
Example: find the height of the plane.
We know the distance to the airplane is 1000
And the angle is 60°
What is the aeroplane'due south top?
Careful! The 60° angle is at the meridian, so the "h" side is Adjacent to the angle!
- Pace 1 The two sides we are using are Adjacent (h) and Hypotenuse (1000).
- Stride ii SOHCAHTOA tells united states to use Cosine.
- Step 3 Put our values into the Cosine equation:
cos threescore° = Adjacent / Hypotenuse
= h / chiliad
- Step iv Solve:
Start with: cos lx° = h/1000
Swap: h/thou = cos lx°
Calculate cos 60°: h/m = 0.5
Multiply both sides past g: h = 0.5 x one thousand
h = 500
The peak of the aeroplane = 500 meters
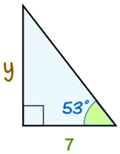
Case: Detect the length of the side y:
- Stride one The two sides nosotros are using are Opposite (y)
and Adjacent (vii).
- Footstep two SOHCAHTOA tells united states to use Tangent.
- Footstep 3 Put our values into the tangent role:
tan 53° = Opposite/Side by side
= y/7
- Step 4 Solve:
Get-go with: tan 53° = y/seven
Bandy: y/seven = tan 53°
Multiply both sides by vii: y = seven tan 53°
Calculate: y = vii 10 1.32704...
y = nine.29 (to 2 decimal places)
Side y = 9.29
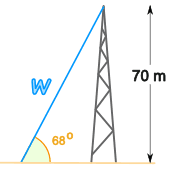
Case: Radio Mast
There is a mast that is seventy meters high.
A wire goes to the top of the mast at an angle of 68°.
How long is the wire?
- Stride ane The two sides we are using are Opposite (seventy) and Hypotenuse (w).
- Step ii SOHCAHTOA tells usa to use Southine.
- Step iii Write down:
sin 68° = 70/due west
- Step 4 Solve:
The unknown length is on the lesser (the denominator) of the fraction!
And then we demand to follow a slightly different approach when solving:
Starting time with: sin 68° = 70/w
Multiply both sides by w: westward × (sin 68°) = 70
Divide both sides past "sin 68°": w = 70 / (sin 68°)
Calculate: w = 70 / 0.9271...
w = 75.v m (to 1 place)
The length of the wire = 75.5 m
Source: https://www.mathsisfun.com/algebra/trig-finding-side-right-triangle.html
Posted by: fraileyallose38.blogspot.com


0 Response to "How To Find One Side Of A Triangle"
Post a Comment